Seminar october 18 of 2023
Seminar in EDP and Applied Mathematics
October 18, 2023 – 14h (Brazil)
Streaming: Youtube Channel | SEMINARIO DE EDP E MATEMATICA APLICADA
Event

About Seminars
Our Online Seminar is one of the most important events in Brazil, it has been held since August 2020, every Wednesday at 2 pm, Brasília time, with a frequency of 14 days. Two 40-minute lectures are presented in each session. Our speakers are world-renowned mathematicians from Europe, the United States and South America.
Featured Talks & Speakers

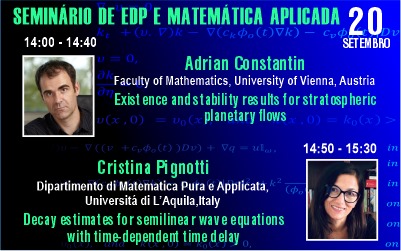
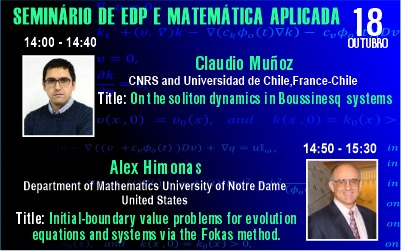
Claudio Muñoz
CNRS and Universidad de Chile,France-Chile
14:00h – 14:40h
On the soliton dynamics in Boussinesq systems
In this talk, I will review recent results in collaboration with several authors on the soliton dynamics in Boussinesq systems, including abcd, improved, good, and the fourth-order phi 4 model. The idea is to show and explain how long time behavior is obtained by using well-defined virial identities.

Alex Himonas
Department of Mathematics University of Notre Dame-United States
14:50h – 15:30h
Initial-boundary value problems for evolution equations and systems via the Fokas method
About Organization
Juan Limaco – UFF – Brazil (Coordenador);
Mauro Rincon – UFRJ – Brazil
Max Souza – UFF – Brazil;
Sandra Malta – LNCC – Brazil
Marcelo Cavalcanti – UEM – Brazil;
Rui Almeida – UBI – Portugal
Roxana Lopez – UNMSM – Peru;
Diego Souza – U Sevilla – España;
Mauricio Sepulveda – UdeC – Chile;
Roberto Capistrano – UFPE – Brazil.
Our Partners








Access our channel!
A channel for students, professors, researchers and professionals who wish to deepen their knowledge in EDP and applied mathematics.